立方形密鋪
外观
(重定向自立方形堆砌)
此條目需要精通或熟悉相关主题的编者参与及协助编辑。 (2014年4月29日) |
 正方形鑲嵌. 1種顏色 |
 立方體堆砌在正圖形的形式 1種顏色 |
 棋盤正方形鑲嵌 2種顏色 |
 立方體堆砌. 2種顏色 |
 擴展正方形鑲嵌 3種顏色 |
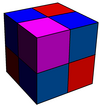 擴展立方體堆砌 4種顏色 |
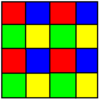 4種顏色 |
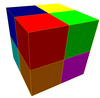 8種顏色 |
在幾何學中,立方形密鋪是一個正密鋪家族,在n維空間中,施萊夫利符號以{4,3...3,4}表示,且n>=3時具有考克斯特群Rn (or B~n-1)的對稱性。
所有的立方形密鋪都屬於自身對偶
考克斯特將在n維空間種的立方形密鋪命名為δn+1以表示其維度。
立方形密鋪在不同維度時有不同稱呼,「密鋪」是指緊密填滿空間。二維時稱做正方形鑲嵌;三維時稱立方體堆砌;四維以上則稱為堆砌或蜂巢體(英語:honeycomb)。
參考文獻
[编辑]- Coxeter, H.S.M. Regular Polytopes, (3rd edition, 1973), Dover edition, ISBN 0-486-61480-8
- pp. 122-123, 1973. (The lattice of hypercubes γn form the cubic honeycombs, δn+1)
- pp. 154-156: Partial truncation or alternation, represented by h prefix: h{4,4}={4,4}; h{4,3,4}={31,1,4}, h{4,3,3,4}={3,3,4,3}
- p. 296, Table II: Regular honeycombs, δn+1
