// **********
// Constantes et initialisation
// **********
clear;
clf;
chdir('monchemin/')
// Paramètres de Newton-Raphson
precision = 1e-7; // condition d'arrêt
itermax = 60; // idem
// Précision de la linéarisation approchée
epsilon = 1e-6;
// **********
// Fonctions
// **********
exec('fonctions_communes.sce', -1)
function [e] = res(Yexp, Ycal)
e = sqrt(sum((Yexp-Ycal).^2));
endfunction
function [A, R] = gaussnewton(f, X, Yexp, A0, imax, epsilon)
// A : jeu de paramètres optimisé par régression (vecteur)
// R : liste des facteurs de qualité de la régression
// pour chaque étape (vecteur)
// X : variable explicative (vecteur)
// Yexp : variable expliquée, valeurs mesurées (vecteur)
// A0 : paramètres d'initialisation du modèle (vecteur)
// epsilon : valeur d'arrêt (scalaire)
k = 1; // facteur d'amortissement initial, <=1,
// évite la divergence
n = size(X,'*');
e0 = sqrt(sum(Yexp.^2)); // normalisation du facteur de qualité
Ycal = f(A0, X); // modèle initial
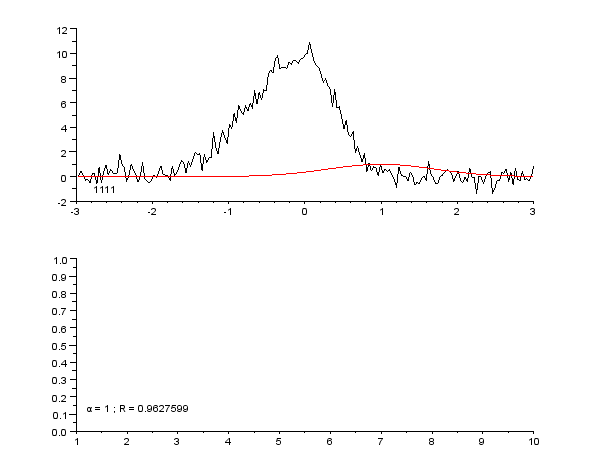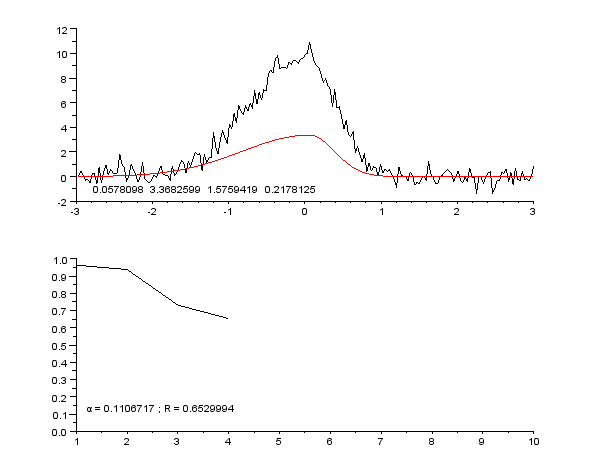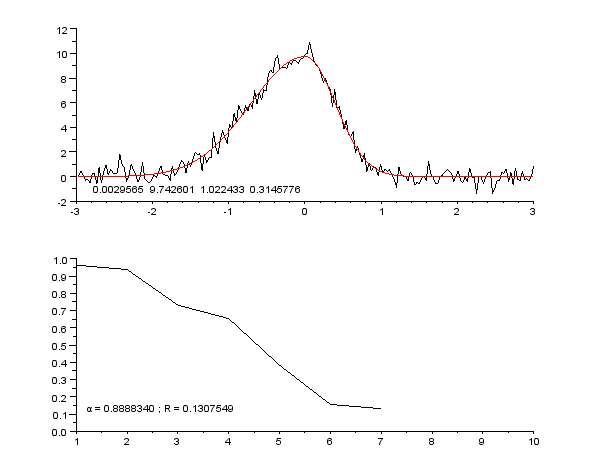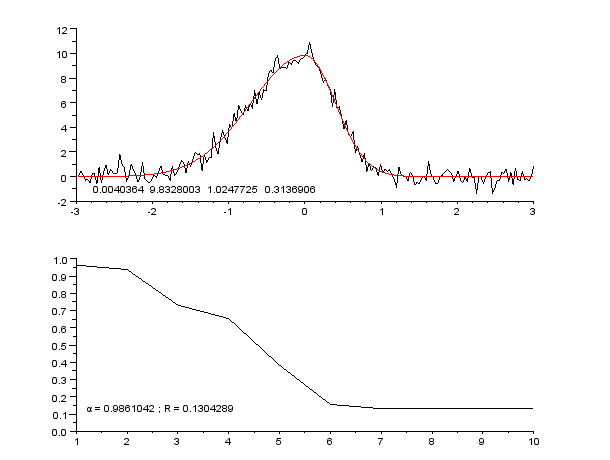
R(1) = res(Yexp, Ycal)/e0; // facteur de qualité initial
disp('i = 1 ; k = 1 ; R = '+string(R(1))) // affichage param initiaux
i = 1;
B = A0;
subplot(2,1,1)
plot2d(X, Yexp, rect=[-3, -2, 3, 12])
plot(X, Ycal, "-r")
xstring(-2.8, -1.5, string(B))
subplot(2,1,2)
plot2d(R, rect=[1, 0, 10, 1])
xstring(1.2, 0.1, 'α = '+string(k)+' ; R = '+string(R(i)))
nom = 'picassym'+string(i)+'.gif';
xs2gif(0,nom)
drapeau = %t;
while (i < imax) & drapeau // teste la convergence globale
i = i+1;
deltay = Yexp - Ycal;
J = linearisation_approchee(f, B, X, epsilon); // matrice jacobienne
tJ = J'; // transposée
deltap0 = inv((tJ*J))*tJ*deltay;
drapeau2 = %t // pour une 1re exécution
while drapeau2 & (k>0.1) // teste la divergence sur 1 étape
deltap = k*deltap0;
Bnouveau = B + deltap';
Ycal = f(Bnouveau, X);
R(i) = res(Yexp, Ycal)/e0;
drapeau2 = (R(i) >= R(i-1)) // vrai si diverge
if drapeau2 then k = k*0.75; // atténue si diverge
else k0 = k; // pour affichage de la valeur
k = (1 + k)/2; // réduit l'atténuation si converge
end
end
B = Bnouveau;
drapeau = abs(R(i-1) - R(i)) > epsilon
clf;
subplot(2,1,1)
plot2d(X, Yexp, rect=[-3, -2, 3, 12])
plot(X, Ycal, "-r")
xstring(-2.8, -1.5, string(B))
subplot(2,1,2)
plot2d(R, rect=[1, 0, 10, 1])
xstring(1.2, 0.1, 'α = '+string(k0)+' ; R = '+string(R(i)))
nom = 'picassym'+string(i)+'.gif';
xs2gif(0,nom)
// disp('i = '+string(i)+' ; k = '+string(k0)+' ; R = '+string(R(i)))
end
A = B;
endfunction
// **********
// Programme principal
// **********
// lecture des données
donnees = read('pic_gauss_dissym_bruite.txt',-1,2);
// carcatéristiques des données
Xdef = donnees(:,1);
Ydef = donnees(:,2);
// Ainit = [-0.03, 9.7, 8*((0.84 - 0.03)/2.35)^2, 8*((0.45 + 0.03)/2.35)^2];
Ainit = [1, 1, 1, 1];
// Régression
tic();
[Aopt, Rnr] =...
gaussnewton(gauss_dissym, Xdef, Ydef,...
Ainit, itermax, precision)
t = toc();
// Courbe calculée
Yopt = gauss_dissym(Aopt, Xdef);
// Affichage
print(%io(2),Ainit)
print(%io(2),Aopt)
print(%io(2),t)
clf
subplot(2,1,1)
plot(Xdef, Ydef, "-b")
plot(Xdef, Yopt, "-r")
subplot(2,1,2)
plot(Rnr)