扭歪無限面體

在幾何學中，扭歪^[1]^[2]無限面體（英語：Skew apeirohedron）是一種頂點並非全部共面的無限面體，存在非平面的面或非平面的頂點圖，並保持圖形不折回形成封閉區間而無限延伸。其也可以看作是面數無法被窮盡的扭歪多面體。由於該多面體所形成的空間有如海綿般有很多孔洞，因此又稱為海綿多面體^[3]。

正扭歪無限面體

關於考克斯特，1926年時，約翰·弗林德斯·皮特里將扭歪多邊形（非平面多邊形）的概念推廣到四維空間的扭歪多面體和三維空間的正扭歪無限面體^[4]。

考克斯特和皮特里發現了三種三維空間的正扭歪無限面體：

扭歪無限面體
{4,6\|4} 多立方體四角六片四角孔扭歪無限面體	{6,4\|4} 多八面體六角四片四角孔扭歪無限面體	{6,6\|3} 多四面體六角六片三角孔扭歪無限面體

戈特的扭歪無限面體

约翰·理查德·戈特在1976年時發表了一個較大的扭歪無限面體系列，該系列共有七種不同的扭歪無限面體，其中也包括了考克斯特和皮特里發現的那三種：{4,6}、{6,4}和{6,6}，另外還多了四種{5,5}、{4,5}、{3,8}、{3,10}^[5]^[6]。

{p,q}	胞	頂點附近的面	圖像	空間群			相關的 H² 軌形記號（英语：Orbifold notation）
{p,q}	胞	頂點附近的面	圖像	立方空間群	考克斯特記號（英语：Coxeter notation）	纖維流形記號（英语：Fibrifold notation）	相關的 H² 軌形記號（英语：Orbifold notation）
{4,5}	立方體			Im3m	$\left[\left[4,3,4\right]\right]$	8^o:2	*4222
{4,5}	截角八面體			I3	$\left[\left[4,3^{+},4\right]\right]$	8⁰:2	2*42
{3,7}	正二十面體			Fd3	$\left[\left[3^{\left[4\right]}\right]\right]^{+}$	2^o−	3222
{3,8}	正八面體			Fd3m	$\left[\left[3^{\left[4\right]}\right]\right]$	2⁺:2	2*32
{3,8}^[7]	扭稜立方體			Fm3m	$\left[4,{\left(3,4\right)}^{+}\right]$	2⁻⁻	32*
{3,9}	正二十面體			I3	$\left[\left[4,3^{+},4\right]\right]$	8⁰:2	22*2
{3,12}	正八面體			Im3m	$\left[\left[4,3,4\right]\right]$	8^o:2	2*32

半正扭歪無限面體

亦存在其他的半正或均勻（點可遞）的扭歪無限面體。瓦茨曼、伯特和克雷曼發現了許多例子^[8]，但他們不知道他們列出的列表是否完整。

半正扭歪無限面體與其相關堆砌
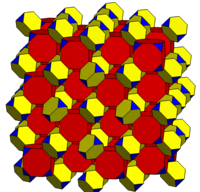
4.4.6.6		6.6.8.8

與大斜方截半立方體堆砌相關，		與施萊夫利符號為h_2,3{4,3,4}的幾何圖形相關，
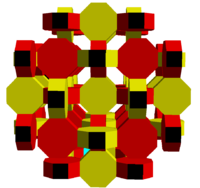
4.4.4.6	4.8.4.8	3.3.3.3.3.3.3

與大斜方截角立方體堆砌相關，
4.4.4.6	4.4.4.8	3.4.4.4.4
		與小斜方截半正方體堆砌（英语：runcitruncated cubic honeycomb）相關，

柱體形半正扭歪無限面體與其相關堆砌
4.4.4.4.4	4.4.4.6
與相關	與相關

一種半正的曲面的幾何結構	堆疊立方體

參見

參考文獻

Coxeter, Regular Polytopes, Third edition, (1973), Dover edition, ISBN 0-486-61480-8
Kaleidoscopes: Selected Writings of H.S.M. Coxeter, edited by F. Arthur Sherk, Peter McMullen, Anthony C. Thompson, Asia Ivic Weiss, Wiley-Interscience Publication, 1995, ISBN 978-0-471-01003-6 [1] （页面存档备份，存于互联网档案馆）
- (Paper 2) H.S.M. Coxeter, "The Regular Sponges, or Skew Polyhedra", Scripta Mathematica 6 (1939) 240-244.
John H. Conway, Heidi Burgiel, Chaim Goodman-Strauss, (2008) The Symmetries of Things, ISBN 978-1-56881-220-5 (Chapter 23, Objects with prime symmetry, pseudo-platonic polyhedra, p340-344)
Schulte, Egon, Chiral polyhedra in ordinary space. I, Discrete and Computational Geometry, 2004, 32 (1): 55–99, MR 2060817, doi:10.1007/s00454-004-0843-x . [2] （页面存档备份，存于互联网档案馆）
A. F. Wells, Three-Dimensional Nets and Polyhedra, Wiley, 1977. [3]
E. Schulte, J.M. Wills On Coxeter's regular skew polyhedra （页面存档备份，存于互联网档案馆）, Discrete Mathematics, Volume 60, June–July 1986, Pages 253–262

^ 400年ぶりに新種の「対称性多面体」構造が発見される. gigazine.net. 2014-02-22 [2016-07-16]. （原始内容存档于2020-11-19）.
^ 扭歪の意味. Weblio日中中日辞典. [2024-04-23]. （原始内容存档于2013-07-20）.
^ Michael Burt- Prof emeritus, Technion, I.I.T. Haifa Israel. Periodic Sponge Surfaces And Uniform Sponge Polyhedra In Nature And In The Realm Of The Theoretically Imaginable. 塞爾維亞科學與藝術學院. [2016-08-19]. （原始内容存档于2020-07-19）.
^ Coxeter, H. S. M. Regular Skew Polyhedra in Three and Four Dimensions. Proc. London Math. Soc. 43, 33-62, 1937.
^ J. R. Gott, Pseudopolyhedrons, American Mathematical Monthly, Vol 74, p. 497-504, 1967.
^ The Symmetries of things, Pseudo-platonic polyhedra, p.340-344
^ Richard Klitzing. Gott's snic-based pseudopolyhedron. 3D convex uniform polyhedra. bendwavy. [2021-10-16]. （原始内容存档于2021-09-30）.
^ A. Wachmann, M. Burt and M. Kleinmann, Infinite polyhedra, Technion, 1974. 2nd Edn. 2005.

外部連結

[1] 400年ぶりに新種の「対称性多面体」構造が発見される. gigazine.net. 2014-02-22 [2016-07-16]. （原始内容存档于2020-11-19）.

[2] 扭歪の意味. Weblio日中中日辞典. [2024-04-23]. （原始内容存档于2013-07-20）.

[3] Michael Burt- Prof emeritus, Technion, I.I.T. Haifa Israel. Periodic Sponge Surfaces And Uniform Sponge Polyhedra In Nature And In The Realm Of The Theoretically Imaginable. 塞爾維亞科學與藝術學院. [2016-08-19]. （原始内容存档于2020-07-19）.

[4] Coxeter, H. S. M. Regular Skew Polyhedra in Three and Four Dimensions. Proc. London Math. Soc. 43, 33-62, 1937.

[5] J. R. Gott, Pseudopolyhedrons, American Mathematical Monthly, Vol 74, p. 497-504, 1967.

[6] The Symmetries of things, Pseudo-platonic polyhedra, p.340-344

[bendwavy_Gott's_snic-based_pseudopolyhedron-7] Richard Klitzing. Gott's snic-based pseudopolyhedron. 3D convex uniform polyhedra. bendwavy. [2021-10-16]. （原始内容存档于2021-09-30）.

[8] A. Wachmann, M. Burt and M. Kleinmann, Infinite polyhedra, Technion, 1974. 2nd Edn. 2005.

[1]

[2]

[3]

[4]

[5]

[6]

[7]

[8]