剪切模量
外觀
| 剪力模數 | |
|---|---|
常見符號 | G, S |
| 國際單位 | 帕斯卡 |
從其他物理量的推衍 | G = τ / γ |
| 因次 | |
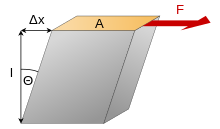
剪力模數(shear modulus)是材料力學中的名詞,彈性材料承受剪應力時會產生剪應變,定義為剪應力與剪應變的比值。公式記為
其中, 表示剪力模數, 表示剪應力, 表示剪應變。在均質且等向性的材料中:
其中, 是楊氏模數(Young's modulus ), 是泊松比(Poisson's ratio)。
波
[編輯]在均勻各向同性固體中,有兩種波:P波和S波。剪切波的速度,由剪切模量控制,
其中
- G是剪切模量
- 是固體的密度.
金屬的剪切模量
[編輯]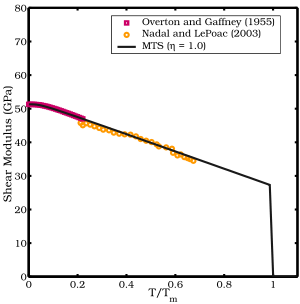
金屬的剪切模量通常隨溫度的升高而降低。在高壓下,剪切模量也隨外加壓力的增大而增大。在許多金屬中,熔點溫度、空位形成能和剪切模量之間的關係已經被觀察到。[3]
有幾種模型試圖預測金屬的剪切模量(可能還有合金的剪切模量)。在塑性流動計算中使用的剪切模量模型包括:
- MTS剪切模量模型由機械閾值應力(MTS)塑性流動應力模型開發並與之結合使用。[4][5][6]
- 由SCGL流動應力模型開發並與之結合使用的SCGL剪切模量模型。[7]
- 納達爾和LePoac (NP)剪切模量模型,利用Lindemann理論確定剪切模量對溫度的依賴關係,利用SCG模型確定剪切模量對壓力的依賴關係。[2]
MTS剪切模型
[編輯]MTS剪切模量模型為:
其中為處的剪切模量,和為材料常數。
SCG剪切模型
[編輯]NP剪切模型
[編輯]剪切鬆弛模量
[編輯]參見
[編輯]| 換算公式 | ||||||||||
|---|---|---|---|---|---|---|---|---|---|---|
| 均質各向同性線彈性材料具有獨特的彈性性質,因此知道彈性模量中的任意兩種,就可由下列換算公式求出其他所有的彈性模量。 | ||||||||||
- ^ Overton, W.; Gaffney, John. Temperature Variation of the Elastic Constants of Cubic Elements. I. Copper. Physical Review. 1955, 98 (4): 969. Bibcode:1955PhRv...98..969O. doi:10.1103/PhysRev.98.969.
- ^ 2.0 2.1 Nadal, Marie-Hélène; Le Poac, Philippe. Continuous model for the shear modulus as a function of pressure and temperature up to the melting point: Analysis and ultrasonic validation. Journal of Applied Physics. 2003, 93 (5): 2472. Bibcode:2003JAP....93.2472N. doi:10.1063/1.1539913.
- ^ March, N. H., (1996), Electron Correlation in Molecules and Condensed Phases (頁面存檔備份,存於網際網路檔案館), Springer, ISBN 0-306-44844-0 p. 363
- ^ Varshni, Y. Temperature Dependence of the Elastic Constants. Physical Review B. 1970, 2 (10): 3952–3958. Bibcode:1970PhRvB...2.3952V. doi:10.1103/PhysRevB.2.3952.
- ^ Chen, Shuh Rong; Gray, George T. Constitutive behavior of tantalum and tantalum-tungsten alloys (PDF). Metallurgical and Materials Transactions A. 1996, 27 (10): 2994 [2019-11-22]. Bibcode:1996MMTA...27.2994C. doi:10.1007/BF02663849. (原始內容存檔 (PDF)於2020-10-01).
- ^ Goto, D. M.; Garrett, R. K.; Bingert, J. F.; Chen, S. R.; Gray, G. T. The mechanical threshold stress constitutive-strength model description of HY-100 steel. Metallurgical and Materials Transactions A. 2000, 31 (8): 1985–1996 [2019-11-22]. doi:10.1007/s11661-000-0226-8. (原始內容存檔於2017-09-25).
- ^ Guinan, M; Steinberg, D. Pressure and temperature derivatives of the isotropic polycrystalline shear modulus for 65 elements. Journal of Physics and Chemistry of Solids. 1974, 35 (11): 1501. Bibcode:1974JPCS...35.1501G. doi:10.1016/S0022-3697(74)80278-7.














































































