跳至內容

星形多面體

維基百科，自由的百科全書

在幾何學中，星形多面體是一種非凸多面體，通常表示外表有如星形一般的多面體[1]，其可以視為星形多邊形之於多邊形在三維空間中的類比。[2]

種類

[編輯]

一般星形多面體可以分為兩種不同的形式：

  • 以重複方式自我相交[3]的多面體。
  • 交替地重複凸與凹或鞍形頂點之凹多面體。在數學中，這種結構是星形域的示例[4]。更白話的說法則是外觀有明顯凸出之頂點的立體[5][6]。

自相交多面體

[編輯]

一般數學上研究的星形多面體通常是正圖形、半正圖形或均勻圖形以及其對偶多面體[7]（如均勻多面體對偶），同時，這些立體通常都會有面自我相交的情況。[8]

星形正多面體

[編輯]
主條目：星形正多面體

星形正多面體是面或邊有與同一立體中其他面或邊相交的情況的正多面體。其通常會具有星形的面或星形的頂點圖，即如星形般相交的一系列面構成的多面角。[1]

星形正多面體

星形均勻多面體

[編輯]

星形均勻多面體是面或邊有與同一立體中其他面或邊相交的情況的均勻多面體。

部分的星形均勻多面體
均勻多面體 對偶多面體

五角星柱（英語：pentagrammic prism）是一種星形均勻柱體（英語：Prismatic uniform polyhedron），由兩個五角星面和5個正方形面組成。
五角星柱的對偶多面體是雙五角星錐（英語：pentagrammic dipyramid），同時也是一種星形多面體，由10個等腰三角形組成。

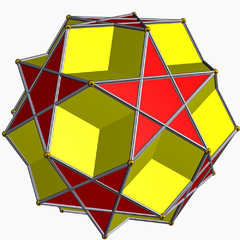截半大十二面體是一種由五邊形和五角星組成的星形均勻多面體[9]
截半大十二面體的對偶多面體為內側菱形三十面體，同時也是一種星形多面體，由30個菱形組成[10]

星形域的多面體

[編輯]
教堂的摩拉維亞星。

一些沒有自相交情況的多面體同樣也可以算是星形多面體，這類多面體通常是交替地重複凸與凹或鞍形頂點之凹多面體，這類多面體是星形域的實例之一[11]。許多自相交的星形多面體皆可以找到一個外觀相同的非自相交多面體，但其在抽象幾何學中具有不同的結構，例如截半大十二面體以及一個不包含內部結構的截半大十二面體[12][13][14]，同時也有可能有2個外觀相同但結構不同的星形多面體，例如內側三角六邊形二十面體與大三角六邊形二十面體[15][16][17][18]。

這種星形多面體通常用於各種建築的裝飾中，尤其在一些宗教建築中[19][20]，例如在巴洛克式教堂、匈牙利教堂和其他宗教建築物上，星形多面體被視為建造教堂的教宗的象徵，同時，也可以在來當作裝飾品，如摩拉維亞星（英語：Moravian star）。[21]

參見

[編輯]

參考文獻

[編輯]
  1. ^ 1.0 1.1 前川淳. 摺紙幾何學：60種特殊摺紙. 科學視界. 世茂出版社. 2018-04-02. ISBN 9789578799165. 
  2. ^ はじめての多面体おりがみ: 考える頭を作ろう. Heart warming life series. 日本ヴォーグ社. 2001: 84. ISBN 9784529035477. 
  3. ^ Paul Bourke; Polygons and meshes:Surface (polygonal) Simplification （頁面存檔備份，存於互聯網檔案館） 1997. (retrieved May 2016)
  4. ^ Ian Stewart, David Tall, Complex Analysis. Cambridge University Press, 1983, ISBN 0-521-28763-4, MR0698076
  5. ^ 心、星、球. etoe.tc.edu.tw. [2019-09-27]. （原始內容存檔於2019-09-27）. 
  6. ^ 巫翌婕, 林欣儀, 賴螢葶, 吳宜真. 凸起而遇－關於星形多面體的研究 (PDF). shs.edu.tw. [2019-09-27]. （原始內容存檔 (PDF)於2019-09-27）. 
  7. ^ Weisstein, Eric W. (編). Star Polyhedron. at MathWorld--A Wolfram Web Resource. Wolfram Research, Inc. （英語）. 
  8. ^ 三村 文武, 岩下 有里. ユニットによる星形多面体の構成 (PDF). 広島経済大學研究論集 (広島経済大學). 2011年9月, 32 (2): 23––34 [2019-09-27]. （原始內容存檔 (PDF)於2019-09-27）. 
  9. ^ David A. Richter. The Dodecadodecahedron and the Golay Code. wmich.edu. [2019-09-27]. （原始內容存檔於2018-10-18）. 
  10. ^ Versi-Regular Polyhedra: Medial Rhombic Triacontahedron. dmccooey.com. （原始內容存檔於2016-03-24）. 
  11. ^ C.R. Smith, A characterization of star-shaped sets, American Mathematical Monthly, Vol. 75, No. 4 (April 1968). p. 386, MR0227724,
    JSTOR 2313423
  12. ^ Geometric Model by Dick Holl, a Student of A.Harry Wheeler, Dodecadodecahedron. americanhistory.si.edu. [2019-09-27]. （原始內容存檔於2019-09-26）. 
  13. ^ Paper Dodecadodecahedron. polyhedra.net. 
  14. ^ Dodecadodecahedron. polyhedr.com. [2019-09-27]. （原始內容存檔於2019-09-26）. 
  15. ^ great triambic icosahedron. bulatov.org. （原始內容存檔於2016-03-03）. 
  16. ^ Weisstein, Eric W. (編). Great Triambic Icosahedron. at MathWorld--A Wolfram Web Resource. Wolfram Research, Inc. （英語）. 
  17. ^ Weisstein, Eric W. (編). Medial Triambic Icosahedron. at MathWorld--A Wolfram Web Resource. Wolfram Research, Inc. （英語）. 
  18. ^ Guy's polyhedra pages. Some lost stellations of the icosahedron. steelpillow. 2006-07-11. （原始內容存檔於2016-03-13）.  Index Number: 303, Precursor: BnGn, Du Val symbol: De2f2
  19. ^ Tarnai, T.; Krähling, J.; and Kabai, S., "Star Polyhedra: from St Mark's Basilica in Venice to Hungarian Protestant Churches." (PDF), Paper ID209 in Proc. of the IASS 2007, Shell and Spatial Structures: Structural Architecture-Towards the Future Looking to the Past. Venice (Italy: University of IUAV), 2007 [永久失效連結]
  20. ^ Tarnai, T.; Krähling, J.; and Kabai, S., "Star Polyhedra on Hungarian Protestant Churches.", In Proc. of the 13th International Conference on Geometry and Graphics (Ed. G. Weiss). Dresden (Germany: Technical University of Dresden, Germany), 2008 
  21. ^ "The Moravian Star". [2007-10-08]. （原始內容存檔於2007-10-08）. 


外部連結

[編輯]
取自 "https://zh.wikipedia.org/w/index.php?title=星形多面體&oldid=79894071"