虫洞

若地面附近有一個蟲洞，它可能是這樣的

蟲洞（英語：Wormhole），又稱愛因斯坦-羅森橋（英語：Einstein—Rosen bridge），是一種連接時空中不同點的理論結構，它基於愛因斯坦場方程的特殊解。

蟲洞可以想像成一條隧道，其兩端位於時空的不同點（可能是不同的位置和時間）。

其名字有「虫咬的洞」的意思，由约翰阿奇巴德惠勒提出，其举例来自如果一只虫要苹果表面移动，比起沿着表面爬，不如直接从果肉穿过距离更短。

蟲洞與廣義相對論是相容的，但蟲洞是否真的存在還有待觀察。許多科學家假設蟲洞只是第四空間維度的投影，類似於二維生物如何只能體驗三維物體的一部分 理論上，一個蟲洞可能連接十億光年這樣的極遠距離，也可能連接幾米這樣的短距離，或者不同的時間點，甚至不同的宇宙。

1995年，馬特·維瑟提出，如果在早期宇宙中生成負質量的宇宙弦，宇宙中可能存在許多蟲洞。 一些物理學家，例如法蘭克·迪普勒和基普·索恩，提出了如何人工製造蟲洞。

宇宙間的旅行

由蟲洞啟用的時間旅行引起的悖論的可能解決方案取決於量子力學的多世界解釋。

1991年大衛·多伊奇表明量子理論在具有閉合類時曲線的時空中是完全一致的（意思是密度矩陣可以被做成沒有不連續） 然而，後來表明，這種閉合類時曲線模型可能存在內部的不一致，因為它會導致奇怪的現象，像是區分非正交量子態和區分適當和不適當的混合態。 因此半經典計算的結果表明了虛擬粒子在蟲洞時間機器中循環的破壞性正反饋迴路是不可能的。從未來返回的粒子不會返回其起源的宇宙，而是返回平行宇宙。這表示，理論上，具有極短時間跳躍的蟲洞時間機器是同時的兩個平行宇宙之間的橋樑。

因為蟲洞時間機器在量子理論中引入了一種非線性項，平行宇宙之間的這種通信與约瑟夫·波尔钦斯基提出的“埃弗里特電話”一致（在史蒂文·溫伯格的非線性量子力學表述中，依休·艾弗雷特三世的名字命名）

平行宇宙之間交流的可能性被稱為“宇宙間的旅行”。

蟲洞也可以用史瓦西黑洞的彭罗斯图來描繪。在彭罗斯图中，一個運動速度超過光速的物體將可穿過黑洞，並從另一端出現，進入不同的空間、時間或宇宙。這將是一個宇宙間蟲洞。

度規

“蟲洞度規”描述了蟲洞的時空幾何，並作為時間旅行的理論模型。一個（可穿越的）蟲洞度規的例子如下：

$ds^{2}=-c^{2}\,dt^{2}+d\ell ^{2}+(k^{2}+\ell ^{2})(d\theta ^{2}+\sin ^{2}\theta \,d\varphi ^{2}),$

由埃利斯首次提出，此為埃利斯排水孔的特例

一種不可穿越的蟲洞的度規是史瓦西解（見第一張圖）：

$ds^{2}=-c^{2}\left(1-{\frac {2GM}{rc^{2}}}\right)\,dt^{2}+{\frac {dr^{2}}{1-{\frac {2GM}{rc^{2}}}}}+r^{2}(d\theta ^{2}+\sin ^{2}\theta \,d\varphi ^{2}).$

1935年7月發表的一篇文章描述了最初的愛因斯坦-羅森橋。

對於史瓦西球對稱靜態解：

$ds^{2}=-{\frac {1}{1-{\frac {2m}{r}}}}\,dr^{2}-r^{2}(d\theta ^{2}+\sin ^{2}\theta \,d\varphi ^{2})+\left(1-{\frac {2m}{r}}\right)\,dt^{2},$

在這裡$ds$是原時而光速$c=1$

利用$u^{2}=r-2m$，把$r$換成$u$

$ds^{2}=-4(u^{2}+2m)\,du^{2}-(u^{2}+2m)^{2}(d\theta ^{2}+\sin ^{2}\theta \,d\varphi ^{2})+{\frac {u^{2}}{u^{2}+2m}}\,dt^{2}$

數學上將這個解的四維時空想成兩個全等部分，或是兩片紙片，根據 $u>0$ 或 $u<0$決定這個點在哪片時空部分上。這兩個時空部分被$r=2m$ （即 $u=0$）這個超曲面連接。我們稱這樣的連接是個「橋」

——愛因斯坦、羅森：「廣義相對論中的粒子問題」

考慮到重力場以及電場，愛因斯坦和羅森推導出以下史瓦西靜態球對稱解

$\varphi _{1}=\varphi _{2}=\varphi _{3}=0,\varphi _{4}={\frac {\varepsilon }{4}},$

$ds^{2}=-{\frac {1}{\left(1-{\frac {2m}{r}}-{\frac {\varepsilon ^{2}}{2r^{2}}}\right)}}\,dr^{2}-r^{2}(d\theta ^{2}+\sin ^{2}\theta \,d\varphi ^{2})+\left(1-{\frac {2m}{r}}-{\frac {\varepsilon ^{2}}{2r^{2}}}\right)\,dt^{2},$

$\varepsilon$ 代表電荷

沒有分母的場方程（在$m=0$的情況下）可寫為

$\varphi _{\mu \nu }=\varphi _{\mu ,\nu }-\varphi _{\nu ,\mu }$

$g^{2}\varphi _{\mu \nu ;\sigma }g^{\nu \sigma }=0$

$g^{2}(R_{ik}+\varphi _{i\alpha }\varphi _{k}^{\alpha }-{\frac {1}{4}}g_{ik}\varphi _{\alpha \beta }\varphi ^{\alpha \beta })=0$

為了去除奇點，若把$r$換為$u$根據：

$u^{2}=r^{2}-{\frac {\varepsilon ^{2}}{2}}$

又$m=0$，可得

$\varphi _{1}=\varphi _{2}=\varphi _{3}=0$ and $\varphi _{4}={\frac {\varepsilon }{\left(u^{2}+{\frac {\varepsilon ^{2}}{2}}\right)^{1/2}}}$

$ds^{2}=-du^{2}-\left(u^{2}+{\frac {\varepsilon ^{2}}{2}}\right)(d\theta ^{2}+\sin ^{2}\theta \,d\varphi ^{2})+\left({\frac {2u^{2}}{2u^{2}+\varepsilon ^{2}}}\right)\,dt^{2}$

在這個解中，對於兩張紙空間中的所有有限點，解都沒有奇點

——愛因斯坦，羅森：「廣義相對論中的粒子問題」

可視化

被視覺化為2D的蟲洞

另一種蟲洞在二維環境的模擬

蟲洞的概念簡單來說，可先將空間想成一種二維表面，而蟲洞就是表面的一個洞，會通過一個管子，然後連結到在二維表面上的另一個位置。由於空間實際上是3維的，所以不同於二維的洞是個圓，入口通道的「洞」其實是一個球。

想像蟲洞的另一種方法是拿一張紙，在紙的一面畫兩個距離稍遠的點。這張紙代表時空連續體中的一個平面。如果把這張紙對折，並建立連接這兩點的橋，走這個橋的距離會比走紙上的距離短得多。這個橋就是蟲洞。

可穿越蟲洞

参见：ER=EPR

洛伦兹蟲洞（史瓦西蟲洞）的電腦繪圖

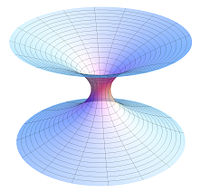
卡西米爾效應指出，量子場論允許空間某些區域的能量密度相對於普通物質的真空能量為負，並且已經從理論上表明，量子場論允許能量在給定點可以任意為負的狀態。 許多物理學家，例如史蒂芬霍金， 基普·索恩，以及其他人， 認為這種效應使穩定可穿越蟲洞成為可能。倫納德·蘇斯金德在他的ER=EPR猜想中提出了理論上，在廣義相對論和量子力學的背景下形成蟲洞的唯一已知自然過程。量子泡沫假說有時被用來表明微小的蟲洞可能會在普朗克尺度上自發出現和消失。穩定的這種蟲洞被視為是暗物質的一種可能選項。 也有人提出，如果在宇宙大霹靂時出現了一個由負質量宇宙弦打開的微小蟲洞，它可能已經因宇宙膨脹，擴張到宏觀尺寸。

洛倫茲可穿越蟲洞將允許從宇宙的一部分非常快速地旅行到同一宇宙的另一部分（而且可以雙向通行），或者允許從一個宇宙旅行到另一個宇宙。荷馬·埃利斯和K·A·布隆尼科夫分別在1973年發表論文，首次證明了廣義相對論中可穿越蟲洞的可能性 埃利斯分析了埃利斯排水孔的拓撲結構和測地線，表明它在測地線上是完整、無事件視界、無奇點的，並且可以在兩個方向上完全穿越。埃利斯排水孔是愛因斯坦真空時空場方程的解流形，由包含最小耦合到具有反正統極性（負而不是正）的里奇張量的純量場修改而成。（因為反正統的耦合，埃利斯特別拒絕將純量場稱為「奇特的」，認為這樣稱呼的論據沒有說服力。）解決方案取決於兩個參數：m，它固定其引力場的強度，n，它決定其空間橫截面的曲率 。當m 設置為 0 時，排水孔的重力場消失。剩下的就是埃利斯蟲洞，一個無引力、純幾何、可穿越的蟲洞。

流行文化

• 蟲洞是科幻小說中的常見元素，因為它們允許星際、星系間，有時甚至是人類生命尺度內的宇宙間旅行。在小說中，蟲洞也被用作時間旅行的一種方法。

• 2014年科幻電影《星際效應》

參考資料

引用

• DeBenedictis, Andrew and Das, A. On a General Class of Wormhole Geometries. arXiv eprint server. [2005-08-12]. （原始内容存档于2017-01-12）. 
• Dzhunushaliev, Vladimir. Strings in the Einstein's paradigm of matter. arXiv eprint server. [2005-08-12]. （原始内容存档于2017-01-12）. 
• Einstein, Albert and Rosen, Nathan. The Particle Problem in the General Theory of Relativity. Physical Review 48, 73 (1935).
• Fuller, Robert W. and Wheeler, John A.. Causality and Multiply-Connected Space-Time. Physical Review 128, 919 (1962).
• Garattini, Remo. How Spacetime Foam modifies the brick wall. arXiv eprint server. [2005-08-12]. （原始内容存档于2017-01-12）. 
• González-Díaz, Pedro F. Quantum time machine. arXiv eprint server. [2005-08-12]. （原始内容存档于2017-01-12）. 
• González-Díaz, Pedro F. Ringholes and closed timelike curves. arXiv eprint server. [2005-08-12]. （原始内容存档于2017-01-12）. 
• Khatsymosky, Vladimir M. Towards possibility of self-maintained vacuum traversable wormhole. arXiv eprint server. [2005-08-12]. （原始内容存档于2017-01-12）. 
• Krasnikov, Serguei. Counter example to a quantum inequality. arXiv eprint server. [2005-08-12]. （原始内容存档于2017-01-12）. 
• Krasnikov, Serguei. The quantum inequalities do not forbid spacetime shortcuts. arXiv eprint server. [2005-08-12]. （原始内容存档于2016-01-26）. 
• Li, Li-Xin. Two Open Universes Connected by a Wormhole: Exact Solutions. arXiv eprint server. [2005-08-12]. （原始内容存档于2017-01-12）. 
• Morris, Michael S., Thorne, Kip S., and Yurtsever, Ulvi. Wormholes, Time Machines, and the Weak Energy Condition. Physical Review Letters 61, 1446–1449 (1988).
• Morris, Michael S. and Thorne, Kip S.. Wormholes in spacetime and their use for interstellar travel: A tool for teaching general relativity Archive.is的存檔，存档日期2012-12-09. American Journal of Physics 56, 395-412 (1988).
• Nandi, Kamal K. and Zhang, Yuan-Zhong. A Quantum Constraint for the Physical Viability of Classical Traversable Lorentzian Wormholes. arXiv eprint server. [2005-08-12]. （原始内容存档于2017-07-02）. 
• Ori, Amos. A new time-machine model with compact vacuum core. arXiv eprint server. [2005-08-12]. （原始内容存档于2014-09-13）. 
• Roman, Thomas, A. Some Thoughts on Energy Conditions and Wormholes. arXiv eprint server. [2005-08-12]. （原始内容存档于2017-01-12）. 
• Teo, Edward. Rotating traversable wormholes. arXiv eprint server. [2005-08-12]. （原始内容存档于2017-01-12）. 
• Visser, Matt. The quantum physics of chronology protection by Matt Visser.. arXiv eprint server. [2005-08-12]. （原始内容存档于2013-09-21）.  An excellent and more concise review.
• Visser, Matt. Traversable wormholes: Some simple examples. Physical Review D 39, 3182–3184 (1989).

外部連結

• 香港太空館：甚麼是“蟲洞”？
• 香港太空馆：黑洞：從幻想到現實
• Creating a Traversable Wormhole（页面存档备份，存于互联网档案馆） by Mohammad Mansouryar
• What exactly is a 'wormhole'? answered by Richard F. Holman, William A. Hiscock and Matt Visser.
• Why wormholes? （页面存档备份，存于互联网档案馆） by Matt Visser.
• Wormholes in General Relativity by Soshichi Uchii.
• New Improved Wormholes （页面存档备份，存于互联网档案馆） by John G. Cramer
• White holes and Wormholes provides a very good description of Schwarzschild wormholes with graphics and animations, by Andrew J. S. Hamilton.
• Wormhole on arxiv.org （页面存档备份，存于互联网档案馆）
• The Big Question: Is time travel possible, and is there any chance that it will ever take place?（页面存档备份，存于互联网档案馆） - Information about the theory the Large Hadron Collider could create a small wormhole. Possibly making time travel into the past possible.
• animation that simulates traversing a wormhole（页面存档备份，存于互联网档案馆）