弦函数

  提示：此条目页的主题不是正弦函数或馀弦函数。

  此条目页的主题是一个三角函数。关于几何学上的弦，请见“弦 (几何)”。

弦函数的函数图形

弦函数（chord function），又称全弦，是最早的三角函数之一，符号通常表示为$\operatorname {crd} \theta$，由古希腊数学家喜帕恰斯所定义，在三角学的早期发展中被广泛使用，主要用于解决天文学计算的问题，现已鲜少使用，但部分的程式库仍会提供弦函数的计算函式。 弦函数的函数值为该角在单位圆上的弦长或圆上特定圆心角$\theta$对应的弦与半径的比值，换句话说，就是单位圆上角的终边端点到始边端点的距离。 弦函数与正弦函数不太一样，但关系十分密切。 在0到π弧度（180度）之间的全弦（crd）与正弦（sin）的关系为crd θ = 2 sin θ/2。

定义

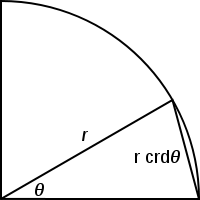
假设角θ介于0和π弧度（180度）之间，则$\operatorname {crd} \theta$的值由圆心角∠AOB构造等腰三角形ΔOAB的底边长${\overline {AB}}$给出，其中O为圆心，即圆心角的顶点。 弦函数的几何定义如右图所示。 角的弦函数值是单位圆上由该圆心角分隔的两点之间的弦的长度。 角度θ取正值，必须位于0 < θ ≤ π（以弧度为单位，或0 < θ ≤ 180度）区间内。 弦函数可以与现代的正弦函数连结起来，取其中一点为(1,0)，另一点为(cos θ, sin θ)，然后利用勾股定理即可计算弦长度。

$\operatorname {crd} \ \theta ={\sqrt {(1-\cos \theta )^{2}+\sin ^{2}\theta }}={\sqrt {2-2\cos \theta }}=2\sin \left({\frac {\theta }{2}}\right).$

弦函数（蓝色）、正弦函数（黄色）与正矢函数（绿色）的函数图形

crd函数（灰色）在单位圆上的位置

原始的弦函数只定义在0至π（180度）之间，可以透过与他对应的等式以及同界角的性质进行扩展定义。图为使用公式$y=\operatorname {crd} \ x={\sqrt {2-2\cos x}}$绘制的弦函数图形

也就是说，弦长度可以透过下列等式来计算：

弦长 = $r\operatorname {crd} \theta =2r\sin {\frac {\theta }{2}}$

其中，r为圆的半径、θ为弦对应的圆心角角度。

弦函数与正弦函数相关联。 在下表中，弦函数可以满足许多类似于众所周知的现代函数的恒等式：

明成	基于正弦函数	基于弦函数
勾股定理	$\sin ^{2}\theta +\cos ^{2}\theta =1\,$	$\operatorname {crd} ^{2}\theta +\operatorname {crd} ^{2}(\pi -\theta )=4\,$
半角公式	$\sin {\frac {\theta }{2}}=\pm {\sqrt {\frac {1-\cos \theta }{2}}}\,$	$\operatorname {crd} \ {\frac {\theta }{2}}={\sqrt {2-\operatorname {crd} (\pi -\theta )}}\,$
边心距 (a)	$c=2{\sqrt {r^{2}-a^{2}}}$	$c={\sqrt {D^{2}-4a^{2}}}$
角 (θ)	$c=2r\sin \left({\frac {\theta }{2}}\right)$	$c={\frac {D}{2}}\operatorname {crd} \ \theta$
其中，c为半径r（直径D）的圆之圆心角θ对应的弦长。

弦函数也可以表达成如下指数定义：

$\operatorname {crd} \ x={\sqrt {2-e^{ix}-e^{-ix}}}$

历史

最早已知的弦函数表由喜帕恰斯编制，其列出了每7+1/2度的弦函数值。 在公元二世纪，亚历山大的托勒密在他的天文学书《天文学大成》中编制了弦函数的函数表——托勒密全弦表，其给出了从1/2度到180度的角度的弦函数值，表中的每行以1/2度为单位。 但其并非是直接以单位圆列出弦函数值，其列出的数值，参考的圆形直径为120，弦长精确到整数部分后两位60进位的数字， 也就是说，托勒密全弦表所列出的值是弦函数的60倍，例如$\operatorname {crd} 60^{\circ }=1$，而在托勒密全弦表中，60度角所记录的值为弦的全长——60。

弦函数与现代常用的正弦函数之关系可以看做是正弦函数代入半角公式的结果。

$\operatorname {crd} \ \theta =2\sin \left({\frac {\theta }{2}}\right)$

上述等式只成立于0 < θ ≤ π（以弧度为单位，或0 < θ ≤ 180度）。

正如现代三角学是建立在正弦函数的基础上一样，古代三角学也是建立在和弦函数的基础上。 据说喜帕恰斯写了一本十二卷的关于弦函数的著作，虽然现在全部都失传了，但想必人们对弦函数有一定的了解。

托勒密的弦函数

主条目：托勒密全弦表

托勒密所建立的托勒密全弦表是纪录特定圆心角θ°在直径120（半径60）的圆形上所对应的弦之长度，换句话说，这个函数表所对应的函数$\operatorname {chord} (\theta )$与正弦函数的关系为：

${\begin{aligned}&\operatorname {chord} (\theta )=120\sin \left({\frac {\theta ^{\circ }}{2}}\right)\\={}&60\cdot \left(2\,\sin \left({\frac {\pi \theta ^{\circ }}{360}}{\text{ radians}}\right)\right).\end{aligned}}$

恒等式

导数

${\frac {\mathrm {d} }{\mathrm {d} \theta }}\operatorname {crd} (\theta )=\cos \left({\frac {\theta }{2}}\right)$，（0 < θ ≤ π）

${\frac {\mathrm {d} }{\mathrm {d} \theta }}\operatorname {crd} (\theta )={\frac {\sin(\theta )}{\sqrt {2-2\cos(\theta )}}}={\frac {2\sin(\theta )(1-\cos(\theta ))+2\sin(\theta )\cos(\theta )}{2{\sqrt {\sin ^{2}(\theta )+(1-\cos(\theta ))^{2}}}}}$

积分

$\int \operatorname {crd} (\theta )\,\mathrm {d} \theta =-4\cos \left({\frac {\theta }{2}}\right)+C$，（0 < θ ≤ π）

$\int \operatorname {crd} (\theta )\,\mathrm {d} \theta =-2{\sqrt {2-2\cos(\theta )}}\cot \left({\frac {\theta }{2}}\right)+C$

其他恒等式

$\operatorname {crd} \left(\alpha \pm \beta \right)={\frac {1}{2}}\operatorname {crd} \left(\alpha \right){\sqrt {4-\operatorname {crd} ^{2}\left(\beta \right)}}\pm {\frac {1}{2}}\operatorname {crd} \left(\beta \right){\sqrt {4-\operatorname {crd} ^{2}\left(\alpha \right)}}$

$\operatorname {crd} \left({\frac {\theta }{2}}\right)={\sqrt {2-\operatorname {crd} (\pi -\theta )}}$

$\operatorname {crd} \left(2\theta \right)=\operatorname {crd} \left(\theta \right){\sqrt {4-\operatorname {crd} ^{2}(\theta )}}=2\sin \theta$

$\operatorname {crd} \left(3\theta \right)=3\operatorname {crd} \left(\theta \right)-\operatorname {crd} ^{3}\left(\theta \right)$

正弦定理也可以写成基于弦函数的形式：

${\frac {\operatorname {crd} \ 2A}{a}}={\frac {\operatorname {crd} \ 2B}{b}}={\frac {\operatorname {crd} \ 2C}{c}}$

其中，a、b和c为三角形的三条边；而A、B和C则为对应边的对角。

与其他三角函数的关系

弦函数可以透过正弦、馀弦和正矢等函数来构造：

$\operatorname {crd} \ \theta =2\sin \left({\frac {\theta }{2}}\right)$，0 < θ ≤ π（0 < θ ≤ 180°）

$\operatorname {crd} \ \theta ={\sqrt {2-2\cos \theta }}$

$\operatorname {crd} \ \theta ={\sqrt {2\operatorname {versin} \ \theta }}$

特殊值

$\operatorname {crd} \left(0\right)=0$

$\operatorname {crd} \left({\frac {\pi }{15}}\right)=\operatorname {crd} \left(12^{\circ }\right)={\frac {{\sqrt {30-6{\sqrt {5}}}}-{\sqrt {6+2{\sqrt {5}}}}}{4}}$

$\operatorname {crd} \left({\frac {\pi }{5}}\right)=\operatorname {crd} \left(36^{\circ }\right)={\frac {{\sqrt {5}}-1}{2}}$

$\operatorname {crd} \left({\frac {\pi }{3}}\right)=\operatorname {crd} \left(60^{\circ }\right)=1$

$\operatorname {crd} \left({\frac {2\pi }{5}}\right)=\operatorname {crd} \left(72^{\circ }\right)={\frac {10-2{\sqrt {5}}}{2}}$

$\operatorname {crd} \left({\frac {\pi }{2}}\right)=\operatorname {crd} \left(90^{\circ }\right)={\sqrt {2}}$

$\operatorname {crd} \left({\frac {3\pi }{5}}\right)=\operatorname {crd} \left(108^{\circ }\right)={\frac {6+2{\sqrt {5}}}{2}}$

$\operatorname {crd} \left({\frac {2\pi }{3}}\right)=\operatorname {crd} \left(120^{\circ }\right)={\sqrt {3}}$

$\operatorname {crd} \left({\frac {4\pi }{5}}\right)=\operatorname {crd} \left(144^{\circ }\right)={\frac {10+2{\sqrt {5}}}{2}}$

$\operatorname {crd} \left(\pi \right)=\operatorname {crd} \left(180^{\circ }\right)=2$

反函数

弦函数的反函数

弦函数的反函数可以定义如下：

$\operatorname {crd} ^{-1}\ y=2\arcsin {\frac {y}{2}}$

在这定义下只有0 ≤ y ≤ 2是有意义的（弦长没有负值、单位圆的弦长不会超过两倍半径）。

或者用$\operatorname {crd} \ \theta ={\sqrt {2-2\cos \theta }}$回推得到：

$\operatorname {crd} ^{-1}\ y=\arccos {\frac {2-y^{2}}{2}}$

反弦函数有时会简称为acrd。

因此已知弦长可以回推圆心角的角度：

$\theta =2\arcsin {\frac {c}{2r}}$

其中，其中c是弦长、r是圆的半径。

相关函数

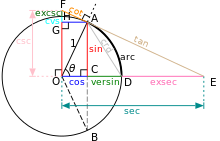
正弦（sin）、馀弦（cos）、全弦（crd）、正矢（versin）、和半正矢（haversin）函数的函数图形

弦函数的值域范围在0到2之间，类似的函数还有正矢函数（versin），值域范围也在0到2之间，但函数图形略有差异。 弦函数在范围0到π（180度）之间的图形与正弦函数0到π/2（90度）的形状类似，但边长差了1倍的缩放倍率。弦函数与其他“正”的三角函数（正弦、正切、正割、正矢）同样是从零开始递增的函数。

arc θ

主条目：弧

弧长（arc θ）、弦长（crd θ）和正弦（sin θ）关系

与弦函数（crd θ）类似的还有另一个符号——arc θ，用于表达指定角θ对应的圆弧之弧长，这个概念有时称为正弧，对应于割圆八线中正角的弧。 早期为了计算天文学上的球面几何问题，例如计算球面三角形和四边形。 因此需要找出arc θ和crd θ的对应关系。在单位圆上，arc θ以黑色较粗的线标示在下图上。

arc θ（黑色较粗之弧线）和crd θ（灰色）、在单位圆上的位置

在单位圆上，arc θ的值在范围0到2π（360度）之间与θ相等，在2π以上时，则为最小正同界角的弧度值。

正弧与馀弧

割圆八线。正角对应的弧为正弧（乙丙）、馀角对应的弧为馀弧（乙庚）

相同的概念在割圆八线中也存在。 arc θ在割圆八线中对应“正弧”，即正角（θ）对应的弧。在这概念下，除了正弧（arc θ）外，也存在对应的馀弧（coarc θ），即馀角对应的弧，等价于直角与正角之差所对应的弧（coarc θ = arc(π/2 − θ)）。 正弧与馀弧的概念早在清代就已经记载于梅文鼎的著作《平三角举要》中了， 并且正弧和馀弧的概念与正弦、馀弦、正切、馀切、正割、馀割、正矢和馀矢并列列出，同时也给角“正角”和“馀角”的概念。

参见

• 三角函数
• 弦 (几何)