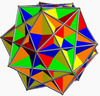
大双三斜三十二面体
外观
 | |||
| 类别 | 均匀星形多面体 | ||
|---|---|---|---|
| 对偶多面体 | 大三角六边形二十面体 | ||
| 识别 | |||
| 名称 | 大双三斜三十二面体 | ||
| 参考索引 | U47, C61, W87 | ||
| 鲍尔斯缩写 | gidtid | ||
| 数学表示法 | |||
| 威佐夫符号 | 3/2 | 3 5 3 | 3/2 5 3 | 3 5/4 3/2 | 3/2 5/4 | ||
| 性质 | |||
| 面 | 32 | ||
| 边 | 60 | ||
| 顶点 | 20 | ||
| 欧拉特征数 | F=32, E=60, V=20 (χ=-8) | ||
| 组成与布局 | |||
| 面的种类 | 20个正三角形{3} 12个正五边形{5} | ||
| 面的布局 | 20{3}+12{5} | ||
| 顶点图 | ((3.5)3)/2 | ||
| 对称性 | |||
| 对称群 | Ih, [5,3], *532 | ||
| 图像 | |||
| |||
在几何学中,大双三斜三十二面体是非凸均匀多面体中的一种星形多面体,其索引编号在均匀多面体中为U47、温尼尔的多面体模型中为W87[1][2]。大双三斜三十二面体的对偶多面体为大三角六边形二十面体[3][4]。
性质
[编辑]大双三斜三十二面体共有32个面、60条边和20个顶点[5][6],其32个面中包括了20个三角形和12个五边形。每个顶点都是3个三角形和3个五边形的公共顶点。
其外接球半径为倍的边长[7],巧合地,立方体的外接球半径也是倍的边长。
在施莱夫利符号的扩充表示法中,大双三斜三十二面体可以用 a{5/2,3} 或c{3,5/2}来表示。在考克斯特记号中也能用![]()
![]()
![]()
![]() 或
或![]()
![]()
![]()
![]()
![]() 来表示,其中
来表示,其中![]()
![]()
![]()
![]() 与
与![]()
![]()
![]()
![]()
![]() 是等价的。
是等价的。
相关多面体
[编辑]| a{5,3} | a{5/2,3} | b{5,5/2} |
|---|---|---|
 小双三斜三十二面体 |
 大双三斜三十二面体 |
 双三斜十二面体 |
 正十二面体 (凸包) |
 五复合立方体 |
对偶复合体
[编辑]大三角六边形二十面体与其对偶的复合体为复合大双三斜三十二面体大三角六边形二十面体。其共有52个面、120条边和52个顶点,其尤拉示性数为-16,亏格为9,有20个非凸面,在威佐夫记号中以(3/2 | 3 5)表示[8]。
参见
[编辑]参考文献
[编辑]- ^ Wenninger, Magnus. Polyhedron Models. Cambridge University Press. 1974. ISBN 0-521-09859-9.
- ^ W87 Great Ditrigonal Icosidodecahedron. colinspics.org. [2016-09-01]. (原始内容存档于2016-09-01).
- ^ Eric W. Weisstein. Great Ditrigonal Icosidodecahedron whose Dual is the Great Triambic Icosahedron.. 密歇根州立大学图书馆. [2016-09-01]. (原始内容存档于2014-07-11).
- ^ Weisstein, Eric W. (编). Great Ditrigonal Icosidodecahedron. at MathWorld--A Wolfram Web Resource. Wolfram Research, Inc. (英语).
- ^ great ditrigonal icosidodecahedron. bulatov.org. [2016-09-01]. (原始内容存档于2016-03-26).
- ^ Uniform Polyhedra 47: great ditrigonal icosidodecahedron. mathconsult. [2016-09-01]. (原始内容存档于2016-03-28).
- ^ Weisstein, Eric W, CRC concise encyclopedia of mathematics, Boca Raton: Chapman & Hall/CRC, 2003, ISBN 1-58488-347-2
- ^ compound of great ditrigonal icosidodecahedron and great triambic icosahedron. bulatov.org. [2016-09-01]. (原始内容存档于2016-03-04).



