跳转到内容

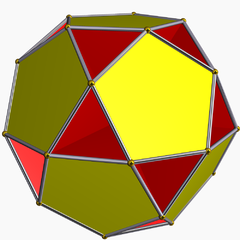
小十二面半十二面体

本页使用了标题或全文手工转换
维基百科，自由的百科全书
  提示：此条目页的主题不是小二十面半十二面体或小十二面半二十面体。
小十二面半十二面体
小十二面半十二面体
类别均匀星形多面体
半多面体
对偶多面体小十二面半无穷星形十二面体（英语：Small dodecahemidodecacron）
识别
名称小十二面半十二面体
Small dodecahemidodecahedron
参考索引U51, C65, W91
鲍尔斯缩写
（verse-and-dimensions的wikia：Bowers acronym）		sidhid
数学表示法
威佐夫符号
（英语：Wythoff symbol）		5/4 5 | 5
性质
面18
边60
顶点30
欧拉特征数F=18, E=60, V=30 （χ=-12）
组成与布局
面的种类12个正五边形
6个正十边形
顶点图5.10.5/4.10
对称性
对称群Ih（英语：Icosahedral symmetry）, [5,3], (*532)
图像
立体图
5.10.5/4.10
（顶点图）

小十二面半无穷星形十二面体（英语：Small dodecahemidodecacron）
（对偶多面体）

小十二面半十二面体是一种拟正半多面体[1]，外观看起来像有深及几何中心的三角形坑洞的截半十二面体[2]:143，最早在1881年由亚伯特·巴杜罗（Albert Badoureau）发现并描述[3]。

性质

[编辑]

小十二面半十二面体由18个面、60条边和30个顶点组成[4]，每个顶点都是2个十边形和2个五边形的公共顶点，并且十边形和五边形依照着十边形、五边形、十边形、五边形的顺序沿着交叉四边形分布[5]，在顶点布局中可以用{10, 5/4, 10, 5}来表示。[4]小十二面半十二面体可以视为基于半多面体的一种刻面结果[6][7]，其对应的原像为截半二十面体，更精确地说，小十二面半十二面体的边和顶点的排列方式皆与截半二十面体相同，差别仅在组成的面之种类不同：小十二面半十二面体由五边形和十边形构成；而截半二十面体由五边形和三角形构成。[8]

构成

[编辑]

小十二面半十二面体向内凹陷部分之结构与小二十面半十二面体相同，并且可透过移除小二十面半十二面体的三角形面并补上五边形面来构造。[2]:143此外若作为一个简单多面体，则这个立体可以视为由12个五边形和20个向内凹陷的三角锥侧面所组成[2]:143，也可以视为由12个五角锥拼凑成的立体。[9][10]

二面角

[编辑]

小十二面半十二面体只有一种二面角，为五边形和十边形的二面角 [11]，其值为五平方根倒数的反余弦值：[12]

顶点座标

[编辑]

小十二面半十二面体的顶点座标与截半二十面体相同，差别仅在于顶点间相连方式的不同[13][14]，因此若小十二面半十二面体几何中心位于原点，且边长为单位长则其顶点座标为：[8][11]

[8]
(±1/2, ±φ/2, ±1 + φ/2)[8]

其中φ是黄金比例，值为。

相关多面体

[编辑]

小十二面半十二面体之边的排列方式与截半二十面体及小二十面半十二面体相同[8]。小十二面半十二面体中，五边形的排列方式与截半二十面体相同；十边形的排列方式与小二十面半十二面体相同。[11]这三个立体的边完全共用，面则部分共用。[15]

参见

[编辑]

参考文献

[编辑]
  1. ^ David I. McCooey. Versi-Regular Polyhedra. dmccooey.com. [2021-09-05]. （原始内容存档于2021-07-30）. 
  2. ^ 2.0 2.1 2.2 Wenninger, M.J. Polyhedron Models. Cambridge University Press. 1974 [2021-09-06]. ISBN 9780521098595. LCCN 69010200. （原始内容存档于2021-08-31）. 
  3. ^ Jean Paul Albert Badoureau. Mémoire sur les Figures Isocèles. Journal de l'École polytechnique. 1881, (49): 47-172. 
  4. ^ 4.0 4.1 Roman E. Maeder. 49: small icosihemidodecahedron. mathconsult.ch. MathConsult AG. 1997 [2021-09-06]. （原始内容存档于2020-02-17）. 
  5. ^ Small Dodecahemidodecahedron. Dynamical Geometry, National Tsing Hua University. 2011 [2021-09-06]. （原始内容存档于2021-09-06）. 
  6. ^ Perry Iv, John J and Perman, Jason A and Zaworotko, Michael J. Design and synthesis of metal--organic frameworks using metal--organic polyhedra as supermolecular building blocks. Chemical Society Reviews (Royal Society of Chemistry). 2009, 38 (5): 1400–1417. 
  7. ^ Vardhan, Harsh and Yusubov, Mekhman and Verpoort, Francis. Self-assembled metal--organic polyhedra: An overview of various applications. Coordination Chemistry Reviews (Elsevier). 2016, 306: 171–194. 
  8. ^ 8.0 8.1 8.2 8.3 8.4 Klitzing, Richard. icosidodecahedron: o3x5o - id. bendwavy.org. [2016-08-30]. （原始内容存档于2016-03-24）. 
  9. ^ Gunilla Borgefor. Uniform polyhedra, part 1. Centre for Image Analysis, Uppsala University, Sweden. [2021-09-06]. （原始内容存档于2021-09-06）. 
  10. ^ Hafner, Izidor. Dissection of small stellated dodecahedron and great stellated dodecahedron to rhombic triacontahedron and hexecontahedron. Visual Mathematics (Mathematical Institute SASA). 2007, (34). 
  11. ^ 11.0 11.1 11.2 Klitzing, Richard. small dodecahemidodecahedron : sidhid. bendwavy.org. [2021-09-06]. （原始内容存档于2021-09-05）. 
  12. ^ David I. McCooey. Versi-Regular Polyhedra: Small Dodecahemidodecahedron. dmccooey.com. [2021-09-05]. （原始内容存档于2019-10-03）. 
  13. ^ Data of Small Dodecahemidodecahedron. dmccooey.com. [2018-10-17]. （原始内容存档于2017-10-31）. 
  14. ^ Data of Icosidodecahedron. dmccooey.com. [2018-10-17]. （原始内容存档于2017-10-31）. 
  15. ^ Klitzing, Richard. id+seihid+sidhid. bendwavy.org. [2021-09-06]. （原始内容存档于2021-09-05）. 

外部链接

[编辑]
检索自“https://zh.wikipedia.org/w/index.php?title=小十二面半十二面體&oldid=75251269”