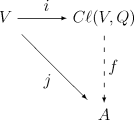克利福德代数:修订间差异
小 調整排版 |
|||
| 第42行: | 第42行: | ||
==泛性質與構造== |
==泛性質與構造== |
||
設<math>V</math>為[[域 (數學)|域]]<math>K</math>上的[[向量空間]],<math>Q: V \to K</math>為<math>V</math>上的[[二次型]]。多數情況下,域<math>K</math>是[[實數|實域]]<math>\mathbb R</math>或[[複數|複域]]<math>\mathbb C</math>,或[[有限域]]<math>\mathbb F_q</math>。 |
|||
克里福代數<math>\mathrm{Cl}(V, Q)</math>定義為有序對<math>(A_0, i)</math>,{{註|{{harv|Vaz|da Rocha|2016}}明確指出映射<math>i</math>(引文作<math>\gamma</math>)是克里福代數結構的一部分,其定義寫作:「有序對<math>(A, \gamma)</math>為二次空間<math>(V, g)</math>的克里福代數,若<math>A</math>作為代數是由<math>\{ \gamma (\boldsymbol v) | \boldsymbol v \in V\}</math>和<math>\{a 1_A | a \in \mathbb R\}</math>生成,且<math>\gamma</math>滿足:對所有<math>\boldsymbol v, \boldsymbol u \in V</math>,有<math>\gamma(\boldsymbol v) \gamma (\boldsymbol u) + \gamma(\boldsymbol u)\gamma (\boldsymbol v) = 2 g(\boldsymbol v, \boldsymbol u)</math>。」}}<ref>{{citation |author=P. Lounesto |title= Counterexamples in Clifford algebras with CLICAL |pages=3–30 |journal=Clifford Algebras with Numeric and Symbolic Computations| year=1996 |doi= 10.1007/978-1-4615-8157-4_1 |isbn= 978-1-4615-8159-8 }},或[https://users.aalto.fi/~ppuska/mirror/Lounesto/counterexamples.htm 刪節版]</ref>其中<math>A_0</math>為<math>K</math>上的[[有單位的|單位]][[結合代數]],而[[線性映射]]<math>i: V \to \mathrm{Cl}(V, Q)</math>滿足對任意<math>v \in V</math>,皆有<math>i(v)^2 = Q(v)1</math>,且<math>(A_0, i)</math>滿足下列[[泛性質]]:給定<math>K</math>上任何單位結合代數<math>A</math>和線性映射<math>j: V \to A</math>令 |
|||
:<math>j(v)^2 = Q(v)1_A \quad \forall v \in V</math> |
|||
(其中<math>1_A</math>表示<math>A</math>的乘法單位元),必有唯一的[[代數同態]]<math>f: \mathrm{Cl}(V, Q) \to A</math>使得以下圖表[[交換圖表|可交換]](即<math>f \circ i = j</math>: |
|||
<div style="text-align: center;">[[Image:CliffordAlgebra-01.png]]</div> |
|||
二次型<math>Q</math>可換成滿足<math>\langle v, v \rangle = Q(v)</math>的(無需對稱的)[[雙線性形式]]<math>\langle \bullet, \bullet \rangle</math>,此時<math>j</math>需滿足的條件等價於 |
|||
:<math> j(v)j(v) = \langle v, v \rangle 1_A \quad \forall v \in V. </math> |
|||
當基域的特徵非<math>2</math>時,以上條件也等價於: |
|||
:<math> j(v)j(w) + j(w)j(v) = ( \langle v, w \rangle + \langle w, v \rangle )1_A \quad \forall v,w \in V , </math> |
|||
其中雙線性型不妨限定為對稱雙線性型。 |
|||
以上描述的克里福代數必定存在,能藉以下一般方法構造:先選取由<math>V</math>生成的最自由的代數,即[[張量代數]]<math>T(V)</math>,然後藉取[[商環|商]],保證基本恆等式成立。對於克里福代數,所需<math>T(V)</math>的雙邊[[理想 (環論)|理想]]<math>I_Q</math>是由所有形如 |
|||
:<math>v\otimes v - Q(v)1</math> |
|||
的元素生成,其中<math>v</math>取遍<math>V</math>的元素,隨後便可定義<math>\mathrm{Cl}(V, Q)</math>為商代數<math>T(V) / I_Q</math>。 |
|||
商承繼的[[環 (數學)|環]]乘積有時稱為'''克里福積'''{{sfn|Lounesto|2001|loc=§1.8}},以免與外代數的外積<math>\wedge</math>或純量積<math>\cdot</math>混淆。 |
|||
It is then straightforward to show that {{nowrap|Cl(''V'', ''Q'')}} contains ''V'' and satisfies the above universal property, so that Cl is unique up to a unique isomorphism; thus one speaks of "the" Clifford algebra {{nowrap|Cl(''V'', ''Q'')}}. It also follows from this construction that ''i'' is [[injective function|injective]]. One usually drops the ''i'' and considers ''V'' as a [[linear subspace]] of {{nowrap|Cl(''V'', ''Q'')}}. |
|||
The universal characterization of the Clifford algebra shows that the construction of {{nowrap|Cl(''V'', ''Q'')}} is ''functorial'' in nature. Namely, Cl can be considered as a [[functor]] from the [[category (mathematics)|category]] of vector spaces with quadratic forms (whose [[morphism]]s are linear maps preserving the quadratic form) to the category of associative algebras. The universal property guarantees that linear maps between vector spaces (preserving the quadratic form) extend uniquely to algebra homomorphisms between the associated Clifford algebras. |
|||
==基與維數== |
==基與維數== |
||
2021年8月5日 (四) 16:48的版本
| 此條目目前正依照en: Clifford algebra上的内容进行翻译。 (2021年8月5日) |
| 此條目需要擴充。 (2015年4月14日) |
| 环论 |
|---|
 |
數學上,克利福德代数(Clifford algebra)是由具有二次型的向量空間生成的單位結合代數。作為域上的代數,其推廣實數系、複數系、四元數系等超複數系,以及外代数。[1][2]此代數結構得名自英國數學家威廉·金顿·克利福德。
研究克里福代数的理論有時也稱為克里福代數,其與二次型論和正交群理論緊密聯繫。其在几何、理論物理、數碼圖像處理中有很多应用。其主要贡献者有:威廉·哈密顿(四元数),赫尔曼·格拉斯曼(外代数),威廉·金顿·克利福德,David Hestenes等。
最常見的克里福代數是正交克里福代數,又稱(偽)黎曼克里福代數。另一類是扭對稱克里福代數。[3]
定義及基本性質
設有域上的向量空間,且其上有二次型。克里福代數是由生成的最自由的單位代數結合代數,但須滿足[註 1]
其中左邊的平方是該代數中的乘法,而右邊的為其乘法單位元。所謂「最自由」,可以用泛性質嚴格定義,詳見下節。
若為有限維實向量空間,且非退化,刖可記為,表示有一組正交基,其中個基元滿足,另有個基元滿足,而指明該克里福代數定義在實域上,即該代數的元素系數皆為實數。此組正交基可藉正交對角化找出。
由生成的自由代數是張量代數。換言之,其為自身的重張量積,對所有的直和。故相應的克里福代數會是該張量代數對元素(取遍的元素)生成的雙邊理想的商。張量積導出在商代數的乘積以串接表示(例如)。其結合律由張量積的結合律推出。
克里福代數有指明的子空間,即嵌入的像。若只得與克里福代數同構的代數,則一般無法唯一確定該子空間。
若底域的特徵不為,則可將基本恆等式重寫成
其中
特徵為的二次型與克里福代數為特例。具體而言,若,則對於二次型,式未必唯一確定某個對稱雙線性型,也未必有正交基。本條目不少命題的條件皆要求特徵不為,而若允許特徵為,則命題不再成立。
作為外代數的量子化
克里福代數與外代數密切相關。外代數是克里福代數的特例:若在克里福代數的定義中,取,則克里福代數就是外代數。即使非零,只要基域的特徵非,和之間仍有典範的線性同構。換言之,兩者作為向量空間自然地同構,但其上的乘法有分別。特徵為時,兩者仍線性同構,然而該同構並非自然。克里福代數的乘法和指定的子空間是比外代數更豐富的結構,因為用到提供的額外資訊。
具體而言,克里福代數可視為外代數的「量子化」(見量子群),正如外爾代數為對稱代數的量子化。
外爾代數和克里福代數還具有*-代數的結構,並能整合成某個超代數的偶次和奇次項,見典範對易與反對易關係代數。
泛性質與構造
設為域上的向量空間,為上的二次型。多數情況下,域是實域或複域,或有限域。
克里福代數定義為有序對,[註 2][4]其中為上的單位結合代數,而線性映射滿足對任意,皆有,且滿足下列泛性質:給定上任何單位結合代數和線性映射令
(其中表示的乘法單位元),必有唯一的代數同態使得以下圖表可交換(即:
二次型可換成滿足的(無需對稱的)雙線性形式,此時需滿足的條件等價於
當基域的特徵非時,以上條件也等價於:
其中雙線性型不妨限定為對稱雙線性型。
以上描述的克里福代數必定存在,能藉以下一般方法構造:先選取由生成的最自由的代數,即張量代數,然後藉取商,保證基本恆等式成立。對於克里福代數,所需的雙邊理想是由所有形如
的元素生成,其中取遍的元素,隨後便可定義為商代數。
商承繼的環乘積有時稱為克里福積[5],以免與外代數的外積或純量積混淆。
It is then straightforward to show that Cl(V, Q) contains V and satisfies the above universal property, so that Cl is unique up to a unique isomorphism; thus one speaks of "the" Clifford algebra Cl(V, Q). It also follows from this construction that i is injective. One usually drops the i and considers V as a linear subspace of Cl(V, Q).
The universal characterization of the Clifford algebra shows that the construction of Cl(V, Q) is functorial in nature. Namely, Cl can be considered as a functor from the category of vector spaces with quadratic forms (whose morphisms are linear maps preserving the quadratic form) to the category of associative algebras. The universal property guarantees that linear maps between vector spaces (preserving the quadratic form) extend uniquely to algebra homomorphisms between the associated Clifford algebras.
基與維數
例子:實域上與複域上的克里福代數
實域上
複域上
註
- ^ 研究實克里福代數且偏好正定二次型者(尤其研究指標理論者),有時在基本克里福恆等式中使用不同的符號。換言之,其取。代為,便可切換兩種約定。
- ^ (Vaz & da Rocha 2016)明確指出映射(引文作)是克里福代數結構的一部分,其定義寫作:「有序對為二次空間的克里福代數,若作為代數是由和生成,且滿足:對所有,有。」
參考資料