在群论 中,循环群 (英文 :cyclic group),是指能由单个元素所生成 群 。有限 循环群同构 于整数 同余 加法群
Z
/
n
Z
{\displaystyle \mathbb {Z} {\big /}n\mathbb {Z} }
同构 于整数加法群。每个循环群都是阿贝尔群 ,亦即其运算是可交换的 。在群论中,循环群的性质已经被研究的较为透彻,是更为复杂的代数研究中常用到的基础工具。
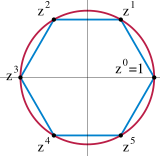
6次单位根 在乘法下形成循环群。z z 2 不是,因为z 幂 不是z 2 的幂。 设
(
G
,
⋅
)
{\displaystyle (G,\cdot )}
g
∈
G
{\displaystyle g\in G}
G
=
⟨
g
⟩
=
{
g
k
|
k
∈
Z
}
{\displaystyle G=\left\langle \,g\,\right\rangle =\left\{g^{k}|\;k\in \mathbb {Z} \right\}}
(
G
,
⋅
)
{\displaystyle (G,\cdot )}
循环群 。群
G
{\displaystyle G}
G
{\displaystyle G}
子群 。
令循环群
G
=
{
g
k
|
k
∈
Z
}
{\displaystyle G=\left\{g^{k}|\;k\in \mathbb {Z} \right\}}
m
,
n
{\displaystyle m,n}
g
m
=
g
n
{\displaystyle g^{m}=g^{n}}
d
=
|
m
−
n
|
{\displaystyle d=\vert m-n\vert }
g
d
=
e
{\displaystyle g^{d}=e}
e
{\displaystyle e}
k
{\displaystyle k}
g
k
=
g
r
{\displaystyle g^{k}=g^{r}}
r
{\displaystyle r}
k
{\displaystyle k}
除以
d
{\displaystyle d}
0
≤
r
≤
d
−
1
{\displaystyle 0\leq r\leq d-1}
G
{\displaystyle G}
有限群 。设
d
m
{\displaystyle d_{m}}
G
{\displaystyle G}
G
=
{
g
k
|
k
=
0
,
1
,
…
,
d
m
−
1
}
{\displaystyle G=\left\{g^{k}|\;k=0,1,\ldots ,d_{m}-1\right\}}
可以证明它同构 于模
d
m
{\displaystyle d_{m}}
(
Z
/
d
m
Z
,
+
)
{\displaystyle \left(\mathbb {Z} {\big /}d_{m}\mathbb {Z} ,+\right)}
n
{\displaystyle n}
同构 的意义上)阶 为此正整数
n
{\displaystyle n}
n
{\displaystyle n}
n
{\displaystyle n}
(
Z
/
n
Z
,
+
)
{\displaystyle \left(\mathbb {Z} {\big /}n\mathbb {Z} ,+\right)}
(
Z
,
+
)
{\displaystyle \left(\mathbb {Z} ,+\right)}
例如,
G
=
{
e
,
g
,
g
2
,
g
3
,
g
4
,
g
5
}
{\displaystyle G=\left\{e,g,g^{2},g^{3},g^{4},g^{5}\right\}}
G
{\displaystyle G}
G
{\displaystyle G}
同构 于模
6
{\displaystyle 6}
Z
6
=
{
0
¯
,
1
¯
,
2
¯
,
3
¯
,
4
¯
,
5
¯
}
{\displaystyle \mathbb {Z} _{6}=\left\{{\overline {0}},{\overline {1}},{\overline {2}},{\overline {3}},{\overline {4}},{\overline {5}}\right\}}
映射 :
φ
:
G
⟶
Z
6
{\displaystyle \varphi :\;G\longrightarrow \mathbb {Z} _{6}}
g
k
↦
k
¯
{\displaystyle \left.\;\,\right.g^{k}\,\mapsto \;\,{\overline {k}}}
可以证明其为群同态 ,而且是双射 ,因此是群同构 。
由于循环群必然是阿贝尔群 ,且与加法群
Z
/
n
Z
{\displaystyle \mathbb {Z} {\big /}n\mathbb {Z} }
Z
{\displaystyle \mathbb {Z} }
Z
n
{\displaystyle \mathbb {Z} _{n}}
p 环 或群的局部化 的标记相冲突,容易混淆。因此,数论中一般直接记作
Z
/
n
Z
{\displaystyle \mathbb {Z} {\big /}n\mathbb {Z} }
C
n
{\displaystyle C_{n}}
每一个循环群要么同构 于整数模
n
{\displaystyle n}
Z
n
=
{
0
¯
,
1
¯
,
2
¯
,
⋯
,
n
−
1
¯
}
{\displaystyle \mathbb {Z} _{n}=\left\{{\overline {0}},{\overline {1}},{\overline {2}},\cdots ,{\overline {n-1}}\right\}}
Z
{\displaystyle \mathbb {Z} }
Z
n
{\displaystyle \mathbb {Z} _{n}}
Z
{\displaystyle \mathbb {Z} }
G
{\displaystyle G}
n
{\displaystyle n}
[ N 1]
g
∈
G
{\displaystyle g\in G}
G
{\displaystyle G}
交换群 。这是因为
g
+
h
≡
h
+
g
(
mod
n
)
{\displaystyle g+h\equiv h+g{\pmod {n}}}
若
n
{\displaystyle n}
g
n
=
e
{\displaystyle g^{n}=e}
n
≡
0
(
mod
n
)
{\displaystyle n\equiv 0{\pmod {n}}}
n
{\displaystyle n}
g
k
=
e
{\displaystyle g^{k}=e}
k
{\displaystyle k}
若
n
{\displaystyle n}
G
{\displaystyle G}
1
{\displaystyle 1}
−
1
{\displaystyle -1}
若
n
{\displaystyle n}
G
{\displaystyle G}
n
{\displaystyle n}
n
{\displaystyle n}
互质 的数的同余类。例如当
n
=
12
{\displaystyle n=12}
G
{\displaystyle G}
Z
12
{\displaystyle \mathbb {Z} _{12}}
1
¯
,
5
¯
,
7
¯
,
11
¯
{\displaystyle {\overline {1}},{\overline {5}},{\overline {7}},{\overline {11}}}
G
{\displaystyle G}
G
{\displaystyle G}
m
{\displaystyle m}
m
{\displaystyle m}
G
{\displaystyle G}
m
Z
{\displaystyle m\mathbb {Z} }
Z
{\displaystyle \mathbb {Z} }
设
p
{\displaystyle p}
素数 ,则阶为
p
{\displaystyle p}
同构 于
p
{\displaystyle p}
两个循环群的直积
Z
n
×
Z
m
{\displaystyle \mathbb {Z} _{n}\times \mathbb {Z} _{m}}
当且仅当
n
{\displaystyle n}
m
{\displaystyle m}
互素 。故
Z
12
{\displaystyle \mathbb {Z} _{12}}
Z
3
×
Z
4
{\displaystyle \mathbb {Z} _{3}\times \mathbb {Z} _{4}}
Z
2
×
Z
6
{\displaystyle \mathbb {Z} _{2}\times \mathbb {Z} _{6}}
[ N 2]
阿贝尔群的基本定理 说明每一个有限生成阿贝尔群 都是有限多个循环群的直积。在二维和三维空间里,
n
{\displaystyle n}
旋转对称 的对称群 为
C
n
{\displaystyle C_{n}}
Z
n
{\displaystyle \mathbb {Z} _{n}}
三维点群 。
需留意的是,圆 的所有旋转所组成之群S 1 (圆群 )不是循环的,甚至不是可数 的。
n
{\displaystyle n}
单位根 形成一个关于乘法的
n
{\displaystyle n}
每一个有限域 之有限扩张 的伽罗瓦群 是有限且循环的;相反地,给定一有限域F 和一有限循环群
G
{\displaystyle G}
F
{\displaystyle F}
G
{\displaystyle G}
有限循环群的环图 全是有着其元素在各个角上的
n
{\displaystyle n}
所有循环群的子群 及商群 都是循环群。特别地,
Z
{\displaystyle \mathbb {Z} }
m
Z
{\displaystyle m\mathbb {Z} }
m
{\displaystyle m}
m
{\displaystyle m}
m
Z
{\displaystyle m\mathbb {Z} }
m
=
0
{\displaystyle m=0}
同构 于
Z
{\displaystyle \mathbb {Z} }
Z
{\displaystyle \mathbb {Z} }
子群格 同构于以可除性 排序之自然数格的对偶 。除了一个平凡的反例
Z
∖
{
0
}
{\displaystyle \mathbb {Z} \backslash \{0\}}
Z
{\displaystyle \mathbb {Z} }
n
{\displaystyle n}
d
{\displaystyle d}
Z
/
n
Z
{\displaystyle \mathbb {Z} /n\mathbb {Z} }
d
{\displaystyle d}
n
/
d
{\displaystyle n/d}
n
{\displaystyle n}
特别地,一个循环群是单群 当且仅当其元素数目为素数。
举一个实际的问题,给定一个
n
{\displaystyle n}
C
{\displaystyle C}
g
{\displaystyle g}
k
{\displaystyle k}
g
k
{\displaystyle g^{k}}
m
{\displaystyle m}
m
{\displaystyle m}
m
k
{\displaystyle mk}
n
{\displaystyle n}
n
/
t
{\displaystyle n/t}
t
{\displaystyle t}
k
{\displaystyle k}
n
{\displaystyle n}
最大公约数 。换句话说,由
g
k
{\displaystyle g^{k}}
指标 为
t
{\displaystyle t}
数论 中被称为指标计算算法
阿贝尔群
Z
n
{\displaystyle \mathbb {Z} _{n}}
自同态环 会同构 于此阿贝尔群,且使其构成一个环 。在此同构之下,数字
r
{\displaystyle r}
n
{\displaystyle n}
Z
n
{\displaystyle \mathbb {Z} _{n}}
r 和n 互素时会是个双射函数,所以
Z
n
{\displaystyle \mathbb {Z} _{n}}
自同构群 会同构于群
Z
n
×
{\displaystyle \mathbb {Z} _{n}^{\times }}
Z
n
{\displaystyle \mathbb {Z} _{n}}
Z
n
{\displaystyle \mathbb {Z} _{n}}
特征群 ,且此一群的建构会直接导致对狄利克雷特征 的定义。
相似地,加法群
Z
{\displaystyle \mathbb {Z} }
Z
{\displaystyle \mathbb {Z} }
Z
{\displaystyle \mathbb {Z} }
{
−
1
,
+
1
}
≅
Z
2
{\displaystyle \{-1,+1\}\cong \mathbb {Z} _{2}}
一个群称为逼肖循环 (virtually cyclic)的,如果这个群包含一个有限指数的循环子群。换言之,一个逼肖循环群的任何元素,都可表示为这个循环子群的一个元素乘以群中某个有限子集的一个元素。一个无限群是逼肖循环的,当且仅当这个群是有限生成 并且正好有两个端 。[ 1]
Z
/
n
{\displaystyle \mathbb {Z} /n}
Z
{\displaystyle \mathbb {Z} }
直积 ,因子
Z
{\displaystyle \mathbb {Z} }
n
{\displaystyle n}
格罗莫夫双曲群 的阿贝尔 子群都是逼肖循环群。[ 2]
^ n n ^
Z
2
{\displaystyle \mathbb {Z} _{2}}
Z
6
{\displaystyle \mathbb {Z} _{6}}
^ Stallings, John, Groups of cohomological dimension one, Applications of Categorical Algebra (Proc. Sympos. Pure Math., Vol. XVIII, New York, 1968), Providence, R.I.: Amer. Math. Soc.: 124–128, 1970, MR 0255689 p. 126 : "If G has two ends, the explicit structure of G is well known: G is an extension of a finite group by either the infinite cyclic group or the infinite dihedral group."^ Alonso, J. M.; Brady, T.; Cooper, D.; Ferlini, V.; Lustig, M.; Mihalik, M.; Shapiro, M.; Short, H., Notes on word hyperbolic groups, Group theory from a geometrical viewpoint (Trieste, 1990) (PDF) , River Edge, NJ: World Scientific, Corollary 3.6, 1991 [2014-04-01 ] , MR 1170363 原始内容 (PDF) 存档于2013-04-25)
Gallian, Joseph, Contemporary abstract algebra 4th, Boston: Houghton Mifflin, 1998, ISBN 978-0-669-86179-2(英语) Herstein, I. N., Abstract algebra 3rd, Prentice Hall , 1996, ISBN 978-0-13-374562-7MR 1375019